We examine Cantor’s diagonal argument for both actual and potential infinity.
• Analysis of Cantor’s diagonal argument for actual and potential infinity [2015/6/26]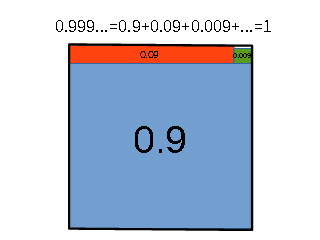
We use the following equation to illustrate actual infinity.
At first glance, one might think the right-hand side is larger than the left. However, the equation is valid, as shown below.
Let us denote the following value by the variable S.
Subtracting S from ten times S gives:
\begin{align*} 10S &= 9.999\cdots \\ -)\quad S &= 0.999\cdots \\ 9S &= 9 \end{align*}Dividing both sides of this equation by nine, we obtain:
Hence, we arrive at:
When I first encountered this expression, I pictured an endless string of 9s. We refer to this unending line of 9s as "actual infinity." Visualizing such an infinite string is quite demanding. Is there a simpler way to understand this equation? To address this, we introduce the concept of limits.
Consider the following sequence:
\begin{align*} a_1 & = 0.9 \\ a_2 & = 0.99 \\ a_3 & = 0.999 \\ a_n & = 0.999\cdots 9 \end{align*}This sequence approaches 1 without ever reaching it:
We call the value that the sequence an never reaches the limit, and denote it using "lim":
$$ \lim_{n \to \infty} a_n = 1 $$We interpret 0.999... not as a standalone number but as the limit:
$$ 0.999\cdots = \lim_{n \to \infty} a_n $$Thus, we observe:
In this view, we need not envision an infinite string of 9s. Instead, we consider the finite sequence 0.9, 0.99, 0.999, and so on, and recognize that it approaches 1, which we then place on the right-hand side of the equation.
We can see this as extending the meaning of the equals sign to relate a number and a sequence that converges to it. This definition introduces no logical contradictions.
In describing limits, we say a sequence approaches a value indefinitely. However, we can formalize this concept more precisely using simpler ideas. This formalization is known as the epsilon-delta definition of a limit.
We write the fact that the sequence an approaches 1 as:
$$ \lim_{n \to \infty} a_n = 1 $$We define the limit rigorously with the epsilon-delta definition as follows:
For every ε > 0, there exists an N such that, for all n > N, |an − 1| < ε holds.
This defines the limit. We can illustrate it with concrete examples:
For ε = 1, choose N = 0. Then for n = 1 (> N), |a1 − 1| < 1. (a1 = 0.9)
For ε = 0.1, choose N = 1. Then for n = 2 (> N), |a2 − 1| < 0.1. (a2 = 0.99)
For ε = 0.01, choose N = 2. Then for n = 3 (> N), |a3 − 1| < 0.01. (a3 = 0.999)
Reading these examples is like playing a game in which the second player always wins: no matter which ε you choose, we can find a suitable N that satisfies the condition.
Although we used ε and N here, the formal definition typically uses ε and δ. Hence, this is called the epsilon-delta definition of a limit.
Since the adjectives "small" and "big" and the adverb "always" are implied, we can omit them for conciseness, yielding the general definition:
For all ε > 0, there exists N such that for all n > N, the inequality |an − 1| < ε holds.
The informal phrasing using terms like "small," "big," and "always" is helpful initially, but with practice we adopt the streamlined formal definition.
Hilbert's Paradox of the Grand Hotel demonstrates the peculiarity of actual infinity. Even if every room in the hotel is occupied, we can accommodate an additional guest by shifting each occupant from room n to room n+1, thereby freeing room 1.
Using his diagonal argument, Cantor proved that the real numbers are uncountable. Details of the proof can be found in the following article.
Now, consider the sequence:
\begin{align*} \pi_1 &= 3.1 \\ \pi_2 &= 3.14 \\ \pi_3 &= 3.141 \end{align*}We then express its limit as:
$$ \pi = \lim_{n \to \infty} \pi_n $$Under actual infinity, π is a fixed constant with an infinite, predetermined decimal expansion.
Under potential infinity, we view π as a function of ε, since the number of digits needed depends on the error bound ε in the epsilon-delta definition of limits.
Zeno’s paradoxes of motion are classic examples illustrating the strangeness of actual infinity.
In order to move from point A to point B, we must first reach the halfway point. To reach that point, we must reach its halfway point, and so on. Thus, reaching B requires passing through infinitely many points, making movement to B impossible.
Under potential infinity, one never reaches point B because the divisions continue indefinitely.
Under actual infinity, we can complete all subdivisions simultaneously and thus reach point B. Now, consider this additional thought experiment:
Each time we reach a subdivision point, we note the next decimal digit in a notebook, erasing the previous entry. Thus, at any moment, the notebook shows only one digit.
If a final digit exists, then t is finite upon arrival at point B. If no final digit exists, what digit remains in the notebook when we reach point B?
However, within the framework of actual infinity, the process is completed simultaneously. Although there is no final digit to record, we still arrive at point B without contradiction.
Infinity refers to either actual infinity or potential infinity. At present, the dominant view in mathematics is actual infinity. Certainly, there is no contradiction in actual infinity. However, I believe that actual infinity does not exist in physical reality.
© 2014, 2015 xseek-qm.net
広告


